A common finding in the educational and psychological measurement literature is the failure of simple IRT models to have good fit for certain items. In other words, an assumption regarding the functional form of the relationship between the latent construct and the item responses is violated. This observation sometimes occurs for psychopathology data, data with mixed populations, and for multiple choice educational tests where there is some effect of guessing. Some alternatives are notorioiusly difficult to estimate (3- and 4-parameter logistic models), and some nonparametric methods may not neatly fit the demands of operational testing programs in which policy and high-stakes decisions are made (e.g., patient-reported outcomes, academic entrance and licensure exams, large-scale international educational assessments). In such testing programs, it is often required that a candidate model is able to be used with multiple groups of participants (such as demographic groups, in order to investigate item or test bias) and planned missing data designs (in which participants do not complete all test items), can be estimated relatively quickly, and can be used in conjunction with a computer adaptive test. In addition, retaining items that would otherwise not fit is highly desirable as such items can cost thousands of dollars to develop. Thus, the overarching goal of this line of research is to develop more flexible modeling approaches that can more readily meet these operational demands.
Flexible and Nonparametric Item Response Models
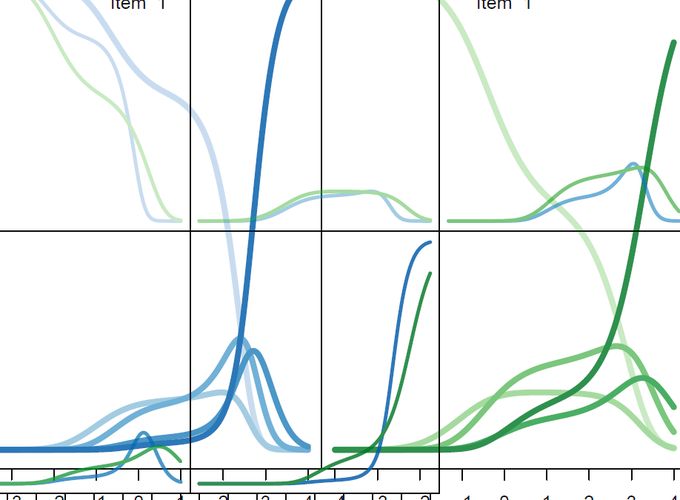
Flexible and Nonparametric Item Response Models
Publications
More flexible response functions for the PROMIS physical functioning item bank by application of a monotonic polynomial approach
Purpose In developing item banks for patient reported outcomes (PROs), nonparametric techniques are often used for investigating …
On the Performance of Semi- and Nonparametric Item Response Functions in Computer Adaptive Tests
Large-scale assessments often use a computer adaptive test (CAT) for selection of items and for scoring respondents. Such tests often …
The Monotonic Polynomial Graded Response Model: Implementation and a Comparative Study
We present a monotonic polynomial graded response (GRMP) model that subsumes the unidimensional graded response model for ordered …
Model Selection for Monotonic Polynomial Item Response Models
One flexible approach for item response modeling involves use of a monotonic polynomial in place of the linear predictor for commonly …
Maximum Marginal Likelihood Estimation of a Monotonic Polynomial Generalized Partial Credit Model with Applications to Multiple Group Analysis
We present a semi-parametric approach to estimating item response functions (IRF) useful when the true IRF does not strictly follow …
Semiparametric Item Response Functions in the Context of Guessing
We present a logistic function of a monotonic polynomial with a lower asymptote, allowing additional flexibility beyond the …